How Ti Find Continuous Time Ear
What is the Effective Annual Rate (EAR)?
Effective annual rate (EAR) is the rate actually earned on investment or paid on the loan after compounding over a given period of time and is used to compare financial products with different compounding periods i.e. weekly, monthly, annually, etc. As the compounding periods are increased, the EAR increases.
Table of contents
- What is the Effective Annual Rate (EAR)?
- Formula
- How to Calculate?
- Example #1
- Example #2
- Example #3
- Conclusion
- Recommended Articles
Formula
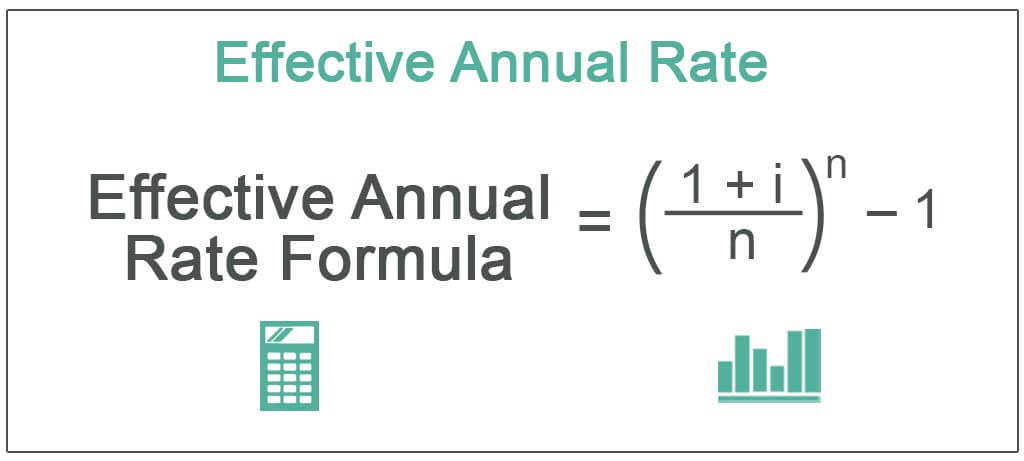
The EAR is calculated as follows:
Effective Annual Rate = (1 + i/n)n – 1
- Where n = number of compounding periods
- i = nominal rate or the given annual rate of interest
You are free to use this image on your website, templates etc, Please provide us with an attribution link
The EAR is equal to the nominal rate only if the compounding is done annually. As the number of compounding periods increase, the EAR increases. If it is continuous compounding formula The continuous compounding formula depicts the interest received when constant compounding is done for an infinite number of periods. The four variables used for its computation are the principal amount, time, interest rate and the number of the compounding period. read more , the EAR is as follows:
Effective Annual Rate (in case of continuous compounding) = ei – 1
Hence, the calculation of the Effective annual rate depends on two factors:
- The nominal rate of interest
- The number of compounding periods
The number of compounding periods is the major factor as the EAR increases with the number of periods.
How to Calculate?
Example #1
Let us consider the following example:
Consider a nominal rate of 12%. Let us calculate the effective annual rate when the compounding is done annually, semi-annually, quarterly, monthly, weekly, daily, and continuously compounded.
Annual Compounding:
- EAR = (1 + 12%/1)1 – 1 = 12%
Semi-Annual Compounding:
- EAR = (1 + 12%/2)2 – 1 = 12.36%
Quarterly Compounding:
- EAR = (1 + 12%/4)4 – 1 = 12.55%
Monthly Compounding:
- EAR = (1 + 12%/12)12 – 1 = 12.68%
Weekly Compounding:
- EAR = (1 + 12%/52)52 – 1 = 12.73%
Daily Compounding:
- EAR = (1 + 12%/365)365 – 1 = 12.747%
Continuous Compounding:
- EAR = e12% – 1 = 12.749%
Thus, as can be seen from the above example, the calculation of the effective annual rate The effective interest rate is the actual rate of interest earned or paid after compounding. It is determined as: Effective Annual Rate Formula = (1 + r/n)n – 1 read more is highest when it is continuously compounded and the lowest when the compounding is done annually.
Example #2
The calculation is important while comparing two different investments. Let us consider the following case.
An investor has $ 10,000, which he can invest in a financial instrument A, which has an annual rate of 10% compounded semi-annually, or he could invest in a financial instrument B, which has an annual rate of 8% compounded monthly. We need to find which financial instrument is better for the investor and why?
To find which instrument is better, we should find the amount he will get after one year from each of the investments:
Amount after one year in Investment A = P * (1 + i/n)n
Where P is the principal, I is the nominal rate, and n is the number of periods of compounding, which is 2 in this case.
- Hence, amount after one year in investment A = 10000 * (1 + 10%/2)2A = $ 11025
Amount after one year in Investment B = P * (1 + i/n)n
Where P is the principal, I is the nominal rate, and n is the number of periods of compounding, which is 12 in this case.
- Hence, amount after one year in investment A = 10000 * (1 + 8%/12)12= B = $ 10830
Thus, in this case, investment A is a better option for the investor since the amount earned after one year is more in investment A.
If the interest is compounded, it results in higher interest in the subsequent periods, the highest being in the last period. Till now, we have considered total amounts at the end of the year.
Example #3
Let us see the following example to find interest at the end of each period.
A financial instrument Financial instruments are certain contracts or documents that act as financial assets such as debentures and bonds, receivables, cash deposits, bank balances, swaps, cap, futures, shares, bills of exchange, forwards, FRA or forward rate agreement, etc. to one organization and as a liability to another organization and are solely taken into use for trading purposes. read more had an initial investment of $ 5000, with an annual rate of 15% compounded quarterly. Let us calculate the quarterly interest received on the investment.
The rate is compounded quarterly The compounding quarterly formula depicts the total interest an investor can earn on investment or financial product if the interest is payable quarterly and reinvested in the scheme. It considers the principal amount, quarterly compounded rate of interest and the number of periods for computation. read more . Hence the interest rate for each quarter = 15%/4 = 3.75%
Interest earned in first quarter = P(1+i/n)n – P = 5000*(1+15%/4) – 5000 = $ 187.5
- Now, the new principal is 5000 + 187.5 = $ 5187.5
Thus, Interest earned in second quarter = P(1+i/n)n – P = 5187.5*(1+15%/4) – 5187.5= $ 194.53
- Now, the new principal is 5187.5+ 194.53 = $ 5382.03
Thus, Interest earned in third quarter = P(1+i/n)n – P = 5382.03*(1+15%/4) – 5382.03= $ 201.82
- Now, the new principal is 5382.03+ 201.82 = $ 5583.85
Thus, Interest earned in fourth quarter = P(1+i/n)n – P = 5583.85*(1+15%/4) – 5583.85= $ 209.39
- Hence, the final amount after one year will be 5583.85 + 209.39 = $ 5793.25
From the above example, we have seen that the interest earned in the fourth quarter is the highest.
Conclusion
The effective annual rate is the actual rate which the investor earns on his investment, or the borrower pays to the lender. It depends on the number of compounding periods and the nominal rate of interest. The EAR increases if the number of compounding periods increases for the same nominal rate, the highest being if the compounding is done continuously.
Recommended Articles
This article has been a guide to the Effective Annual Rate and its definition. Here we also discuss examples of effective annual rate along with its interpretations. You can learn more from the following articles –
- Disadvantages of Nominal Interest Rate
- Effective Interest Rate Calculation
- Formula for Simple Interest
- Simple Interest vs. Compound Interest
escarcegalostower.blogspot.com
Source: https://www.wallstreetmojo.com/effective-annual-rate/
0 Response to "How Ti Find Continuous Time Ear"
Post a Comment